Given the following set of numbers,
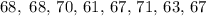
Where the (n) number of data is 8, the mean is,
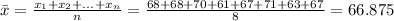
The standard deviation is 3.36
Hence, the interval that is 1 population within the mean is given by
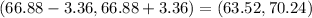
Of all the data only 71, 61, and 63 are not an element of the interval (63.52,70.24)
The total number of data is 8.
Hence, the total number of data within 1 standard deviation of the mean is 5