Question

A woman of mass 58 kg jumps off the bow

of a 52 kg canoe that is intially at rest.
If her velocity is 4.5 m/s to the right, what
is the velocity of the canoe after she jumps?
Answer in units of m/sî.

Answer 1

-5.02 m/s (Assuming the woman's direction is the "positive" direction)

Step-by-step explanation:

The problem is a classic example of conservation of momentum where a woman jumps off a canoe and we need to find the resulting velocity of the canoe. The conservation of momentum dictates that the momentum before and after the jump remains constant, given the system is isolated.

Momentum is calculated using the formula,

`\boxed{\left\begin{array}{ccc}\text{\underline{Momentum:}}\\\\\vec P=m\vec v\\\\\text{Where:}\\\bullet \ \vec P \ \text{is the momentum}\\\bullet \ m \ \text{represents the mass of the object}\\\bullet \ \vec v \ \text{represents the object's velocity}\end{array}\right}`

`\hrulefill`

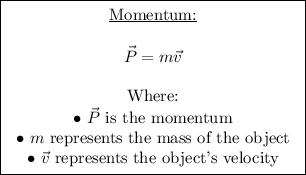
Initial System Momentum: The canoe is initially at rest, and so the initial momentum of the system (woman plus canoe) is zero.

Thus,

`\vec P _0=0 \ (kg \cdot m)/(s)`

Final System Momentum: The momentum gained by the canoe must equal the momentum lost by the woman.

`\Longrightarrow \vec P _f = m_w\vec v_w + m_c\vec v_c\\\\\\\\\Longrightarrow \vec P _f = (58 \ kg)(4.5 \ m/s) + (52 \ kg)\vec v_c`

Using momentum conservation:

`\Longrightarrow \vec P_0=\vec P_f\\\\\\\\\Longrightarrow 0 \ (kg \cdot m)/(s) = (58 \ kg)(4.5 \ m/s) + (52 \ kg)\vec v_c\\\\\\\\\Longrightarrow 0 \ (kg \cdot m)/(s) =261 \ (kg \cdot m)/(s) + (52 \ kg)\vec v_c\\\\\\\\\Longrightarrow 0 \ (kg \cdot m)/(s) - 261 \ (kg \cdot m)/(s) = (52 \ kg)\vec v_c\\\\\\\\\Longrightarrow - 261 \ (kg \cdot m)/(s) = (52 \ kg)\vec v_c\\\\\\\\\Longrightarrow \vec v_c = (- 261 \ (kg \cdot m)/(s))/(52 \ kg) \\\\\\\\`

`\therefore \vec v_c \approx \boxed{-5.02 \ m/s} \text{ (the negative indicates direction)}`

Thus, the canoe is traveling 5.02 m/s opposite the direction of the woman's movement.