Question

Two small triangles ABC and CFJ are to the left and right of bigger triangle CDF. Vertical lines BG, DH, and JI labeled pillars divide the triangles into two. Sides BC, CD, DF, and FJ are labeled guy wire. H is labeled bridge. The diagram shows a section of a bridge between the points A and K. The length of line segment AK is 640 meters. Triangles ABC, CDF, and CFJ are similar, and 2AC = CF = 2FJ. The first pillar is 20 meters tall. The area of triangle CDF is ______ square meters.

Answer 1

The area of triangle CDF is 6400 square meters.

We have similar triangles ABC, CDF, and CFJ, where triangle CDF is the larger middle triangle, and ABC and CFJ are the smaller triangles to the left and right of CDF, respectively. It's given that
`\( 2 * AC = CF = 2 * FJ \)`, which indicates that triangle CDF is twice the height of triangles ABC and CFJ.

Let
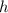`\( h \)` be the height of triangles ABC and CFJ. Therefore, the height of triangle CDF is
`\( 2h \)`, since similar triangles maintain their proportional dimensions.

The height of the first pillar, which we can assume is the height of triangle ABC, is given as 20 meters, so
`\( h = 20 \)` meters.

Now, we need to find the base of triangle CDF. Since
`\( 2AC = CF = 2FJ \)` and triangles ABC, CDF, and CFJ are similar, the bases of these triangles are proportional to their heights. Let's denote the base of triangle ABC as
`\( b \)`. Then the base of triangle CDF would be
`\( 2b \)`, and the base of triangle CFJ would also be
`\( b \)`, making the total base length from A to K equal to
`\( 4b \)`.

Given that AK is 640 meters, we have
`\( 4b = 640 \)` meters. Therefore,
`b = (640)/(4) = 160 \)` meters. The base of triangle CDF, which is twice the base of ABC, is
`\( 2b = 320 \)` meters.

Now we can calculate the area of triangle CDF using the formula for the area of a triangle:

`\[ \text{Area} = (1)/(2) * \text{base} * \text{height} \]`

For triangle CDF:

`\[ \text{Area}_(CDF) = (1)/(2) * 320 * 40 \]`

`\[ \text{Area}_(CDF) = 160 * 40 \]`

`\[ \text{Area}_(CDF) = 6400 \text{ square meters} \]`

The area of triangle CDF is 6400 square meters.