Question

Stephen began a baseball card collection by purchasing some cards. He increase the number of cards in the collection by a constant amount each week after that. The table below shows the total number of cards in the collection at the end of several weeks. Weeks completed since initial purchase :• 3• 6• 11Number of cards in the collection: •285•420•645How many cards did Steve initially purchase to the beginning of his collection?

Answer 1

The situation can be represented by a linear function, which is represented by the following expression:

`\begin{gathered} y=mx+b \\ \text{Where, } \\ m=\text{slope} \\ b=y-\text{intercept} \end{gathered}`

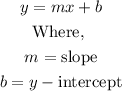
Since he increased the number of cards by a constant amount each week, that means we have proportionality:

`\begin{gathered} ^{}m=(\Delta y)/(\Delta x) \\ m=(420-285)/(6-3) \\ m=(135)/(3)=45 \end{gathered}`

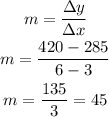
Then, by the slope-point form of the line, we can find the equation and then substitute x=0.

`\begin{gathered} y-y_0=m(x-x_0) \\ y-285=45(x-3) \\ y=45x-135+285 \\ y=45x+150 \end{gathered}`

Substituting, x=0.

`\begin{gathered} y=45(0)+150 \\ y=150 \end{gathered}`

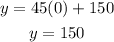
At the beginning of the collection, he has 150 cards.