Question

Two concentric circles are shown. The length of the arc MN is 4π cm and the length of the arc RE is 8π cm.Which statements are correct? Select all that apply.ere to searchThe radius of the larger circle is 16 cm.The radius of the smaller circle is 16 cm.

Answer 1

We can express the length of an arc as a fraction of the circumference.

This fraction is equal to the the proportion between the measure of the arc and the full circle measure.

In this case, the measure is 45°, so the fraction should be:

`\begin{gathered} \alpha=45\degree \\ \Rightarrow f=(\alpha)/(360\degree)=(45\degree)/(360\degree)=0.125 \end{gathered}`

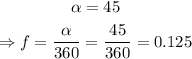
Then, we can express the arc MN as:

`MN=C_1\cdot f`

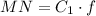
where C1 is the circumference of the smaller circle.

We can express then calculate it as:

`\begin{gathered} MN=C_1\cdot f \\ C_1=(MN)/(f)=(4\pi)/(0.125)=32\pi \end{gathered}`

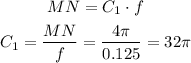
As the circumference can be related to the radius, we can us this value to calcualte the radius of the smaller circle:

`\begin{gathered} C_1=2\pi r_1 \\ r_1=(C_1)/(2\pi)=(32\pi)/(2\pi)=16 \end{gathered}`

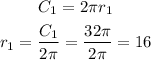
Then, the small circle has a radius of 16 cm and a circumference of 32π cm.

We can then calculate C2 and r2 for the bigger circle as:

`\begin{gathered} RE=C_2\cdot f \\ C_2=(RE)/(f)=(8\pi)/(0.125)=64\pi \end{gathered}`
`\begin{gathered} C_2=2\pi r_2 \\ r_2=(C_2)/(2\pi)=(64\pi)/(2\pi)=32 \end{gathered}`

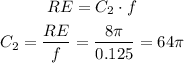
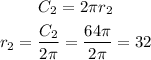
The bigger circle has a radius of 32 cm and a circumference of 64π cm.

We can now calculate the length of the segments NE and MR. Both are equal and can be calculated as the difference between the radius of the bigger circle (r2) and the radius of the smaller circle (r1):

`NE=MR=r_2-r_1=32-16=16`

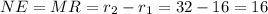
Both segments, NE and MR, have a length of 16 cm.

We can now check the statements. The ones that are true are:

• The radius of the smaller circle is 16 cm.

,

• The length of the segment MR is 16 cm.

,

• The circumference of the larger circle is 64π cm.

Answer: The true statements are

• The radius of the smaller circle is 16 cm.

,

• The length of the segment MR is 16 cm.

• The circumference of the larger circle is 64π cm.