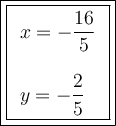Question

Use algebra to find the point at which the line h (x) = -8/3x - 134/15 intersects the line k(x) = 1/3x + 2/3

Write the values of a and y as reduced fractions or integers.

x =
y =

Answer 1

x = -3 and 1/5

y = -2/5

graph is below :)

Answer 2

`x=-(16)/(5)`

`y=-(2)/(5)`

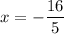
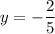
Explanation:

To find the point of intersection, set the two functions equal to each other and solve for the x-value. Then, substitute the x-value into one of the functions and solve for the corresponding y-value.

Set the two functions equal to each other:

`-(8)/(3)x-(134)/(15)=(1)/(3)x+(2)/(3)`

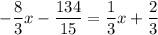
Multiply both sides of the equation by 15 to eliminate the fractions:

`15\left(-(8)/(3)x-(134)/(15)\right)=15\left((1)/(3)x+(2)/(3)\right)`

This simplifies to:

`-40x-134=5x+10`

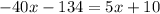
Combine like terms and solve for x:

`-40x-134+40x=5x+10+40x`

`-134=45x+10`

`-134-10=45x+10-10`

`-144=45x`

`(-144)/(45)=(45x)/(45)`

`x=-(144)/(45)`

`x=-(144/9)/(45/9)`

`x=-(16)/(5)`

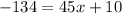
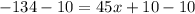
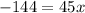
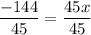
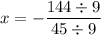
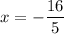
Now, substitute the found x-value back into either equation to find the corresponding y-value. Let's use function k(x):

`y= (1)/(3)\left(-(16)/(5)\right) +(2)/(3)`

`y=-(16)/(15)+(2)/(3)`

`y=-(16)/(15)+(10)/(15)`

`y=-(6)/(15)`

`y=-(6/ 3)/(15/ 3)`

`y=-(2)/(5)`

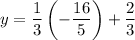
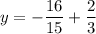
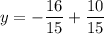
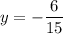
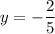
Therefore, the point of intersection is:

`\large\boxed{\boxed{\begin{array}{l}x=-(16)/(5)\\\\y=-(2)/(5)\end{array}}}`