To answer this question we will use the following formula for the arc length of a central angle θ degrees:
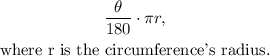
Assuming that Y is the circumference's center we get:
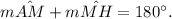
Substituting mMH=88degrees we get:
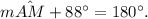
Therefore:
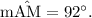
Then the arc length of MA is:
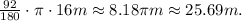
Answer: First option.