Question

How do I do this on a line?
`3 \ \textless \ 2x - 3 \leqslant 13`

Answer 1

Let the inequality:

`3\text{ }<\text{ 2x - 3}\leq\text{ 13}`

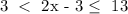
1. we add + 3 :

`3\text{ +3}<\text{ 2x }\leq\text{ 13}+3`

this is equivalent to :

`6<\text{ 2x }\leq\text{ 1}6`

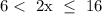
we resolve for x ( we divide by 2) :

`3<\text{ x }\leq8`

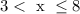
that is the interval:

`(3,\text{ 8}\rbrack`

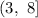
on the real line, the interval is:

On the other hand, the inequality:

`-2\text{ }<(3+x)/(4)\leq\text{ 3}`

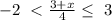
1. Multiply by 4:

`-8\text{ }<3+x\leq12`

2. Add -3:

`-11\text{ }that is the interval:<p></p>[tex](-11,\text{ 9}\rbrack`

on the real line, the interval is: