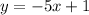Question

Find the equation of the linear function represented by the table below in slope-intercept form.Answer: ?(Important: Please check the attached photo before answering the question)

Answer 1

The Slope-Intercept form of the equation of the line is:

`y=mx+b`

Where "m" is the slope of the line and "b" is the y-intercept.

The slope can be found with:

`m=(y_2-y_1)/(x_2-x_1)`

Choose two points from the table. These could be the points (1,-4) and (4,-19). You can set up that:

`\begin{gathered} y_2=-19 \\ y_1=-4 \\ x_2=4 \\ x_1=1 \end{gathered}`

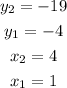
Substituting values, you get that the slope of this line is:

`m=(-19-(-4))/(4-1)=-5`

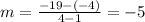
You can substitute the slope and the first point into the equation in Slope-Intercept form:

`-4=1(-5)+b`

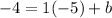
Solve for "b":

`\begin{gathered} -4+5=b \\ b=1 \end{gathered}`

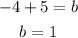
Therefore, the Equation of this line in Slope-Intercept form is:

`y=-5x+1`