Given the function:

Where:
v(t) represents the value of the house after t years.
Let's find the following:
• (a). Find the initial value of the house.
Apply the exponential function:
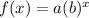
Where:
a is the initial value
b is the growth of decay factor.
Here, we have:
a = 637000
b = 1.02
Therefore, the initial value of the house is 637,000 dollars.
• (b). Does the function represent growth or decay?
If b is greater than 1 the function represents a growth function.
If b is less than 1, the function represents a decay function.
Here, we have:
b = 1.02
Therefore, the function represents a growth function.
• (c). By what percent does the value of the house change each year?
Apply the formula:
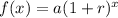
Where r is the growth rate.
Thus, to find r, we have:
1 + r = 1.02
r = 1.02 - 1
r = 0.02
The growth rate is 0.02
To convert the rate to percent multiply by 100:
Growth percent = 0.02 x 100 = 2%
Therefore, the value of the house increases by 2% each year.
ANSWER:
• (a). 637000 dollars
,
• (b). Growth
,
• (c). 2%