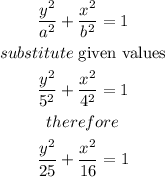we know that
vertices (0,-5) and (0,5) --------> is a vertical ellipse
the minor axis of length 8 ------> 2b=8 -------> b=4
so
Part I: In what direction is this ellipse oriented?
Is a vertical ellipse
Part II: What are the coordinates of the center of this ellipse?
The center of the ellipse is the midpoint between the vertices
The midpoint between (0,-5) and (0,5) is the origin (0,0)
The center is the point (0,0)
Part III: What are the values of a and b for this ellipse?
b=4
2a=10 ---------> a=5
Part IV: Write the equation of this ellipse.