Question

Write an equation of the line in slope intercept form that passes through the given point and is perpendicular to the given line.(-2,4) , y = 2x + 9

Answer 1

We are given the point (-2,4) and the line y=2x+9. We want the equation of the line that passes through the given point and that is perpendicular to the given line.

To do so, we will use the following equation of a line

`y\text{ -a = m\lparen x -b\rparen}`

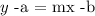
in this equation, m is the slope of the line and (a,b) is a point in the line. In our case, we are given that (-2,4) is in the line. That is, a=-2 and b=4. So our equation becomes

`y\text{ -4=m\lparen x -\lparen-2\rparen\rparen}`

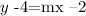
or equivalently

`y\text{ -4}=m(x\text{ +2\rparen}`

now, we only need to find the value of m. To do so, we use the given line and the fact that the product of the slopes of perpendicular lines is -1.

The given line (2x+9) has a slope of 2. So, we have the following equation

`m\cdot2=\text{ -1}`

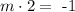
so if we divide both sides by 2, we get that

`m=\text{ -}(1)/(2)`

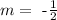
So the equation we are looking for becomes

`y\text{ -4 }=\text{ -}(1)/(2)(x\text{ +2\rparen}`

We want this equation in the slope intercept form. So we operate to find y in this equation. So first, we distribute on the right hand side. We get

`y\text{ -4}=\text{ -}(1)/(2)x\text{ -}(2)/(2)=\text{ -}(1)/(2)x\text{ -1}`

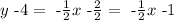
now we add 4 on both sides, so we get

`y=\text{ -}(1)/(2)x\text{ -1+4= -}(1)/(2)x+3`

we can check that if x= -2 we get

`y=\text{ -}(1)/(2)(\text{ -2\rparen+3=1+3=4}`

which confirms that the point (-2,4) is on the line