Answer:
• The number of first-class tickets bought = 3
,
• The number of coach tickets bought =7
Step-by-step explanation:
Let the number of first-class tickets bought = x
Let the number of coach tickets bought = y
A total of 10 people took the trip:
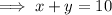
Her total budget for the trip's airfare = $4220.
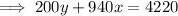
Next, solve the two equations simultaneously:
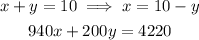
Substitute x into the second equation:
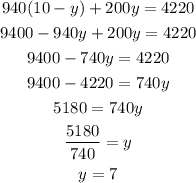
Recall: x=10-y
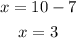
Thus:
• The number of first-class tickets bought, x = 3
,
• The number of coach tickets bought, y =7