Susan is making a pennant in the shape of a triangle for her senior class photo. She wants the base length of this triangle to be 4 inches. The area of the pennant must be at least 12 square inches. (The pennant has to be seen in the photo.) Write an Inequality that describes the possible heights (in inches) of the triangle. Use h for the height of the triangular pennant.
Remember that
the area of the triangle is
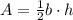
we have
b=4 in
at least -----> is greater than or equal to
so
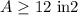
therefore
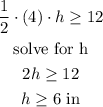
the height of triangle must be greater than or equal to 6 inches