Question

Find the area of quadrilateral ABCD. Round the area to the nearest whole number, if necessary.у| A(-5,4)4B(0, 3)22F(-2,1)-226 xTC(4, -1)-4E(2, -3)D(4, -5)6The area issquare units.

Answer 1

We have a quadrilateral ABCD and we want to calculate the area.

We can divide it in three areas (two triangles and one rectangle) and then add the surfaces.

As it is rotated 45 degrees, we can define a "new unit" that is the diagonal of a square of 1 by 1 unit, in the scale of the graph.

This new unit, the diagonal that we will call "d", by the Pythagorean theorem, has a value of:

`d=√(2)`

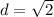
We will start then with the triangle ABF. It has a side BF that has a value of 2 diagonals (2d) and a side FA that has a value of 3 diagonals (3d). The area of a triangle is half the multiplication of this two sides, so we have:

`\frac{\bar{BF}\cdot\bar{FA}}{2}=(2d\cdot3d)/(2)=3d^2=3(√(2))^2=3\cdot2=6`

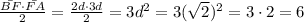
The second triangle is CED. We repeat the process and we have:

`\frac{\bar{CE}\cdot\bar{ED}}{2}=(2d\cdot2d)/(2)=2d^2=2\cdot2=4`

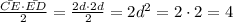
The rectangle BCEF has an area of:

`\bar{BF}\cdot\bar{EF}=2d\cdot4d=8d^2=8\cdot2=16`

Now we have the three areas. If we add them we get the area of ABCD:

`6+4+16=26`

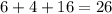
The quadrilateral ABCD has an area of 26 units^2.