Question

How to find the value of X in problem 15

Answer 1

We are asked to determine the value of "x" and "y".

To determine the value of "y" we will use the facto that since WP is a median this means that:

`AP=PH`

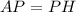
Substituting the values in terms of "y" we get:

`3y+11=7y-5`

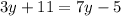
Now, we solve for "y". To do that we will first subtract "7y" from both sides:

`\begin{gathered} 3y-7y+11=7y-7y-5 \\ -4y+11=-5 \end{gathered}`

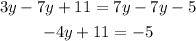
Now, we subtract 11 from both sides:

`\begin{gathered} -4y+11-11=-5-11 \\ -4y=-16 \end{gathered}`

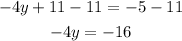
Now, we divide both sides by -4:

`\begin{gathered} y=-(16)/(-4) \\ \\ y=4 \end{gathered}`

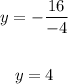
therefore, the value of "y" is 4.

Now, to determine the value of "x" we will use the fact that since WP is an angle bisector we have that:

`m\angle HWP+m\angle PWA=m\angle HWA`

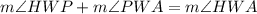
We also have the:

`m\angle PWA=m\angle HWP`

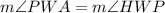
Therefore, we have:

Now, we substitute the values:

`2(x+12)=4x-16`

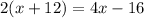
Now, we divide both sides by 2:

`x+12=2x-8`

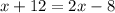
Now, we subtract 2x from both sides:

`\begin{gathered} x-2x+12=2x-2x-8 \\ -x+12=-8 \end{gathered}`

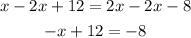
Now, we subtract 12 from both sides:

`\begin{gathered} -x+12-12=-8-12 \\ -x=-20 \\ x=20 \end{gathered}`

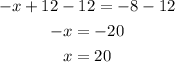
This means that the value of "x" is 20.

To determine if WP is an altitude we need to determine if the angle APW is 90 degrees. To do that we use the fact that the sum of the interior angles of a triangle always adds up to 180, therefore:

`m\angle WPA+m\angle PWA+m\angle PAW=180`

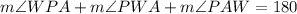
We substitute the values in terms of "x":

`m\angle WPA+(x+12)+(3x-2)=180`

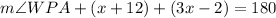
Now, we substitute the value of "x":

`m\angle WPA+(20+12)+(3(20)-2)=180`

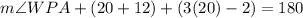
Solving the operations:

`m\angle WPA+90=180`

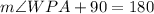
now, we subtract 90 from both sides:

`\begin{gathered} m\angle WPA=180-90 \\ m\angle WPA=90 \end{gathered}`

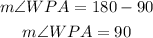
Since WPA is 90 degrees and WP is a median and bisector this means that WP is an altitude.