Question

An automobile battery manufacturer claims that its midgrade battery has amean life of 50 months with a standard deviation of 6 months. Suppose thedistribution of battery lives of this particular brand is approximately normal.ba. On the assumption that the manufacturer's claims are true, find theprobability that a randomly selected battery of this type will last less than 48months.Your answer6b. On the same assumption, find the probability that the mean of a randomsample of 36 such batteries will be less than 48 months.

Answer 1

Given data:

The given mean life is m=50 months.

The given standard deviation is s=6 months.

The given value of battery life is x= 48 months.

The expression for the probability of that a randomly selected battery will last less than 48 months is,

`\begin{gathered} P(Z<(x-m)/(s))=P(Z<(48-50)/(6)) \\ =P(Z<-(1)/(3)) \end{gathered}`

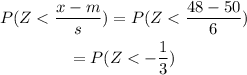
Refere the Z-table the value of the above expression is 0.3707.

Thus, the probability that a randomly selected battery will last less than 48 months is 0.3707.