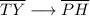Question

100 points
Suppose ΔTYO ≅ ΔPHG. Match the corresponding parts of the triangle

Answer 1

`\angle H \longrightarrow \angle Y`

`\overline{TY} \longrightarrow \overline{PH}`

`\overline{PG} \longrightarrow \overline{TO}`

`\angle O \longrightarrow \angle G`

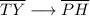
Explanation:

When two triangles are congruent (ΔTYO ≅ ΔPHG), it means that their corresponding sides and angles are equal. To match the corresponding parts of the triangles, we can use the following notation:

ΔTYO corresponds to ΔPHG as follows:

• The side TY corresponds to the side PH.
• The side YO corresponds to the side HG.
• The side TO corresponds to the side PG.

In terms of angles:

• Angle T corresponds to angle P.
• Angle Y corresponds to angle H.
• Angle O corresponds to angle G.

So, these are the corresponding parts of the congruent triangles ΔTYO and ΔPHG.

Therefore, answer is:

`\angle H \longrightarrow \angle Y`

`\overline{TY} \longrightarrow \overline{PH}`

`\overline{PG} \longrightarrow \overline{TO}`

`\angle O \longrightarrow \angle G`