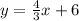Question

Use the point-slope formula to write an equation of the line that passes through (-3, 2) and (-6, -2).Write the answer in slope-intercept form (if possible).

Answer 1

The equation of a line in the slope-intercept form is y = mx + b, where "m" is the slope and b is the y-intercept.

To find the equation of the line given two points (x, y), follow the steps below.

Step 01: Substitute the point (-3, 2) in the equation.

To do it, substitute x by -3 and y by 2.

`\begin{gathered} 2=m\cdot(-3)+b \\ 2=-3m+b \end{gathered}`

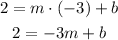
Isolate b by adding 3m to both sides of the equation.

`\begin{gathered} 2+3m=-3m+b-3m \\ 2+3m=-3m+3m+b \\ 2+3m=b \end{gathered}`

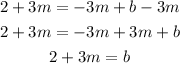
Step 02: Substitute b in the equation of the line.

Knowing that b = 2 + 3m. Then,

`\begin{gathered} y=mx+b \\ y=mx+2+3m \end{gathered}`

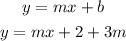
Step 03: Substitute the point (-6, -2) in the equation from step 02.

To do it, substitute x by -6 and y by -2.

`\begin{gathered} -2=m\cdot(-6)+2+3m \\ -2=-6m+2+3m \\ -2=-3m+2 \end{gathered}`

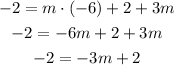
Isolate "m" by subtracting 2 from both sides.

`\begin{gathered} -2-2=-3m+2-2 \\ -4=-3m \end{gathered}`

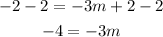
Finally, divide both sides by -3:

`\begin{gathered} (-4)/(-3)=(-3)/(-3)m \\ (4)/(3)=m \end{gathered}`

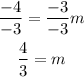
Knowing "m", use the equation from step 1 to find "b".

Step 04: Find "b".

`\begin{gathered} b=2+3m \\ \end{gathered}`

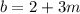
Substituting m by 4/3 and solving the equation:

`\begin{gathered} b=2+3\cdot(4)/(3) \\ b=2+(3\cdot4)/(3) \\ b=2+4 \\ b=6 \end{gathered}`

Answer: The equation of the line is:

`y=(4)/(3)x+6`