Question

I have no idea how to do this but I have to graph and show work step by step. and graph the answer.

Answer 1

Here, we want to graph the given inequality

To do this, we need to get the intercepts of the normal line with the inequality replaced by an equality sign

Thus, we have;

`y\text{ = }(2)/(3)x-1`

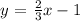
Generally, we have the equation of a linear graph as;

`y\text{ = mx + b}`

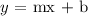
where m represents the slope and b represents the y-intercept

With respect to this question, -1 is y-intercept of the line

Thus, the point of the y-intercept is (0,-1)

Now, we proceed to get the x-intercept.

To do this, we simply substitute the value 0 for y

We have;

`\begin{gathered} 0\text{ = }(2)/(3)x\text{ - 1} \\ \\ (2x)/(3)\text{ = 1} \\ \\ 2x\text{ =3 } \\ \\ x\text{ = }(3)/(2) \\ \\ x\text{ = 1.5} \end{gathered}`

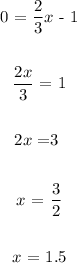
The x-intercept here is thus the point (1.5,0)

Normally, to plot the graph of the line, we simply connect the two intercepts with a straight line

In the case of this inequality too, we are going to join the two points, but this time with dots and not straight lines

And also, since the inequality is greater than, we are simply going to shade the side above the dotted line

Kindly note that if there was an inequality sign, wherein, we have greater than or equal to, we are going to join with a thick line and shade

Let us check what we have in the plot below;