Question

Which of the following equations has roots x=3 (multiplicity 3) and x=−i, and passes through the point (1,-16)?

Answer 1

To verify that a value is a root of a function we use the following setup

F(x) = 0 and replace x by the value in this case 3 and -i.

Let's begin with x=3

`\begin{gathered} f(x)\text{ = }x^3+3x^2+x+3 \\ f(3)\text{ = }3^3+3\cdot3^2+3+3 \\ f(3)\text{ = 60} \end{gathered}`
`\begin{gathered} f(x)\text{ = }x^5+9x^4+28x^3+36x^2+27x+27 \\ f(3)\text{ = 3}^5\text{+9}\cdot3^4\text{{}+28}\cdot3^3\text{+36}\cdot\text{3}^2\text{+27}\cdot3\text{+27} \\ f(3)\text{ = 2160} \end{gathered}`
`\begin{gathered} f(x)\text{ = }x^5-9x^4+28x^3-36x^2+27x-27 \\ f(3)\text{ = 3}^5-\text{9}\cdot3^4\text{{}+28}\cdot3^3-\text{36}\cdot\text{3}^2\text{+27}\cdot3-\text{27} \\ f(3)\text{ = 0} \end{gathered}`
`\begin{gathered} f(x)\text{ = }x^3+3x^2+x+3 \\ f(3)\text{ = }3^3-3\cdot3^2+3-3 \\ f(3)\text{ = 0} \end{gathered}`

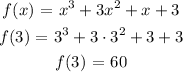
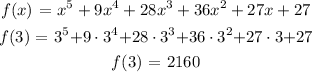
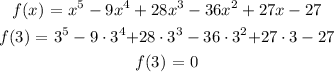
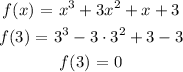
Only options C and D pass the first filter.

Let's apply x=-i to those options

`f(-i)\text{ = }(-i\text{\rparen}^5\text{ - 9}\cdot\left(-i\right)^4\text{ + 28}\cdot\text{\lparen-i\rparen}^3\text{ - 36}\cdot(-i)^2+27\cdot(-i)-27`
`\begin{gathered} f(-i)\text{ = }-i\text{ - 9 + 28 i - 36}-27i-27 \\ f(-i)\text{ = 0} \end{gathered}`
`f(-i)\text{ = \lparen-i\rparen}^3-3\left(-i\right)^2+(-i)-3`
`\begin{gathered} f(-i)\text{ = i+ }3-i-3 \\ f(-i)\text{ = 0} \end{gathered}`

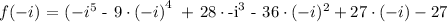
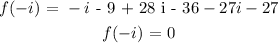
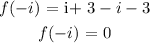
both of the accomplish the equality f(x)=0

So to finish we replace the point to check which functions pass

Replacing f(1) = -16

`\begin{gathered} \left(1\right)^5-9\cdot\left(1\right)^4+28\cdot(1)^3-36^(\cdot)(1)^2+27\cdot(1)-27 \\ f(1)\text{ = -16} \end{gathered}`

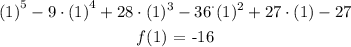
This is equals to -16

`\begin{gathered} \text{ f\lparen1\rparen= 1- }3+1-3 \\ f(1)=-4 \end{gathered}`

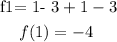
So the answer is option C