To do this, first plug the values of x and y into the given equation. If you get a true statement, the ordered pair will be a solution to the equation, otherwise, it won't.
So, for the ordered pair (2,6) you have
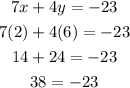
Since the proposition is false, then the ordered pair (2,6) is not a solution to the equation.
For the ordered pair (-5,3) you have

Since the proposition is true, then the ordered pair (-5,3) is a solution to the equation.
For the ordered pair (6,-7) you have
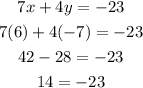
Since the proposition is false, then the ordered pair (6,-7) is not a solution to the equation.
Finally, for the ordered pair (-1,-4) you have

Since the proposition is true, then the ordered pair (-1,-4) is a solution to the equation.