Question

If trapezoid ABCD was dilated by a scale factor of 2\3 to form trapezoid A'B'C'D,what is the area of trapezoid ABCD?The area of trapezoid A'B'C'D is 12 units^2

Answer 1

As a general rule, we know that the area of a dilated figure is the area of the original figure multiplied by the square of the scale factor. We can see this in the following formula:

`A=A^(\prime)\cdot k^2`

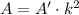
where A is the area of the original figure, A' is the area of the dilated figure and k is the scale factor.

In this case, we have that the area of the dilated figure (trapezoid A'B'C') is 12 square units, and the scale factor is k = 2/3. Then, using the equation we get the following:

`\begin{gathered} A^(\prime)=12 \\ k=(2)/(3) \\ \Rightarrow A=12\cdot((2)/(3))^2=12\cdot((4)/(9))=(12\cdot4)/(9)=(48)/(9)=5(1)/(3) \\ A=5(1)/(3)u^2 \end{gathered}`

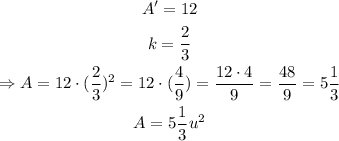
therefore, the area of trapezoid ABCD is 5 1/3 square units