Question

What is the range of the function f(x) = x^2 - 2x -15?*

Answer 1

For this problem, we are given a certain function and we need to determine its range.

The function is:

`f(x)=x^2-2x-15`

This quadratic function has a minimum value in its range, which is represented by its vertex. The best way to determine the range is to find the coordinates of the vertex of this function, therefore we have:

`\begin{gathered} x_(vertex)=(-b)/(2a)=(2)/(2)=1\\ \\ y_(vertex)=f(1)=(1)^2-2(1)-15=1-2-15=-16 \end{gathered}`

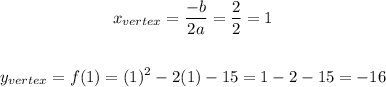
The minimum value in the range is -16, which means that the range is:

`\lbrace f(x)|f(x)\geqslant-16\rbrace`

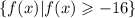
The correct option is the second one.