write the equation for the Connie's pool and Paula's pool
Connie's

y=cubic yards of water remaining in the pool
x=time in seconds
Paula's
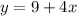
y=cubic yards of water in the pool
x=time in seconds
write the inequality in order for connie's pool to have less water

solve the inequality for x

After 41/7 seconds Connie's pool will have less water than Paula's.