Question

Container A holds 5 times as much milk as Container B. After 1.25 L of milk from Container A is poured into Container B, both containers have the same amount of milk. How much milk does each container have at first?

Answer 1

Container A: 3.125 L

Container B: 0.625 L

Explanation:

Let x represent the starting amount for Container A.

Let y represent the starting amount for Container B.

Set up an equation to represent what is happening.

Note that milk poured out Container A is poured INTO Container B, so the overall value of the equation should remain the same when subtracting 1.25 L from Container A.

`5(y) = x\\x-1.25 = 1.25 + y` →
`x = 2.5 +y`

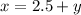
This is now a solvable system of equations. Use substitution to set the equations equal to each other and solve for y.

`5y = 2.5 + y\\4y = 2.5\\y = 0.625 L\\\\x = 5y\\x = 5(0.625)\\x = 3.125 L`

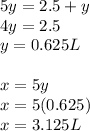
Container A started with 3.125 L and Container B started with 0.625 L.