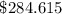Question

Hello, could you help me solve this question? Solve this answer using the sum of the geometric sequence formula.

Answer 1

Given that:

- Cory saves $30 in June.

- Each month he plans to save 10% more than the previous month.

- He saves money from June to December.

You can convert 10% to a decimal number by dividing it by 100:

`10\text{ \%}=(10)/(100)=0.1`

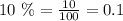
You already know that he has $30 in June. Then, you can determine that the amount of money (in dollars) he will save in July is:

`30+(30\cdot0.1)=33`

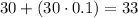
By definition, the formula for the Sum of a Geometric Sequence is:

`S_n=(a_1(1-r^n))/(1-r)`

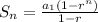
Where "r" is the Common Ratio, "n" is the number of terms, and this is the first term:

`a_1`

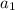
In this case, you can identify that the first term is:

`a_1=30`

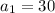
And the second term is:

`a_2=33`

Therefore, you can find the Common Ratio as follows:

`r=(33)/(30)=1.1`

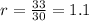
Since he saves money from June to December, the sequence has 7 terms. Then:

`n=7`

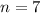
Now you can substitute values into the formula and evaluate:

`S_7=(30(1-(1.1)^7))/(1-r)\approx284.615`

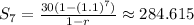
Hence, the answer is:

`\text{ \$}284.615`