Question

Finding the vertex, intercepts, and axis of symmetry from the graph of a parabola

Answer 1

Step-by-step explanation:

Given:

(b) Equation of the axis of symmetry

`\begin{gathered} x=-8 \\ x=4 \end{gathered}`
`\begin{gathered} x=-8,x=4 \\ (x+8)(x-4)=0 \\ x^2-4x+8x-32=0 \\ x^2+4x-32=0 \\ y=x^2+4x-32 \end{gathered}`

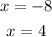
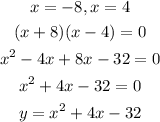
where

`\begin{gathered} y=ax^2+bx+c \\ a=1,b=4,c=-32 \end{gathered}`

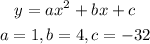
The formula for the axis of symmetry and the x value of the vertex

`x=-(b^2)/(2a)`

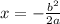
Plug in the value

`x=(-(4)^)/(2)=-2`

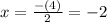
(d) To find the y value of the vertex, substitute 1 for x in the equation.

`\begin{gathered} y=x^2+4x-32 \\ y=(-2)+4(-2)-32 \\ y=-2-8-32 \\ y=-42 \end{gathered}`

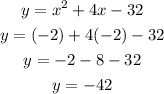
The vertex is (-2 , -42) Since a > 0 the vertex is the minimum point and the parabola opens upward.

Hence the vertex = (-2 , -42)