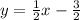Question

1. Write an equation of the line that is parallel to the linewhose equation is 4y + 9 = 2x and passes through thepoint (7,2)

Answer 1

First let's put the equation 4y + 9 = 2x in the slope-intercept form:

`y=mx+b`

Where m is the slope and b is the y-intercept. So we have that:

`\begin{gathered} 4y+9=2x \\ 4y=2x-9 \\ y=(2x-9)/(4) \\ y=(1)/(2)x-(9)/(4) \end{gathered}`

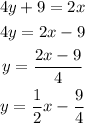
The slope of this equation is 1/2. In order to the second line be parallel to this line, it has to have the same slope. Also, since the second line passes through the point (7, 2), we have:

`\begin{gathered} y=(1)/(2)x+b \\ (7,2)\colon \\ 2=(1)/(2)\cdot7+b \\ 2=(7)/(2)+b \\ b=2-(7)/(2)=(4-7)/(2)=-(3)/(2) \end{gathered}`

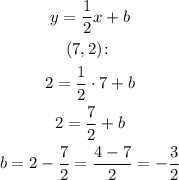
So the second equation is:

`y=(1)/(2)x-(3)/(2)`