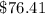Question

Diane opened a savings account 3 years ago the account earns 9% interest compounded monthly if the current balance is 100.00 how much did she despoit initially

Answer 1

The amount she deposited initially is $76.41.

`\text{ \$76.41}`

Step-by-step explanation:

We want to find the amount of Principal she deposited initially.

Recall that the formula for calculating the Principal of a compound interest is;

`P=(A)/((1+(r)/(n))^(nt))`

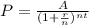
Where;

P = Principal / initial amount

A = Future/ final amount

r = Interest rate (decimal)

n = number of times the interest is compounded per year.

t = Time in years

From the question, we were given the following;

A = $100.00

r = 9% = 0.09

n = monthly (12 months in a year) = 12 times

t = 3 years

Substituting the given values into the formula, we have;

`\begin{gathered} P=(100.00)/((1+(0.09)/(12))^(12(3))) \\ P=(100.00)/((1+0.0075)^(36)) \\ P=(100.00)/((1.0075)^(36)) \\ P=76.41 \\ P=\text{ \$76.41} \end{gathered}`

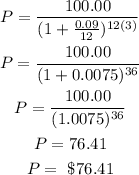
Therefore, the amount she deposited initially is $76.41.

`\text{ \$76.41}`