Question

Consider the equation: x2 – 3x = 18A) First, use the "completing the square" process to write this equation in the form (x + D)² =or (2 – D)? = E. Enter the values of D and E as reduced fractions or integers.=z? - 3x = 18 is equivalent to:– 3rPreview left side of egn:B) Solve your equation and enter your answers below as a list of numbers, separated with a commawhere necessary.Answer(s):

Answer 1

Part A.

The quadratic equation,

`ax^2+bx+c=0`

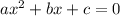
is equivalent to

`a(x+(b)/(2a))^2=(b^2)/(4a)-c`

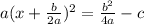
In our case a=1, b=-3 and c=-18. Then, by substituting these value into the last result, we have

`(x+(-3)/(2(1)))^2=((-3)/(2(1)))^2+18`

which gives

`\begin{gathered} (x-(3)/(2))^2=(9)/(4)+18 \\ (x-(3)/(2))^2=(9)/(4)+18 \\ (x-(3)/(2))^2=(9+72)/(4) \\ (x-(3)/(2))^2=(81)/(4) \end{gathered}`

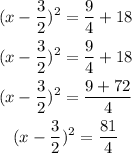
Therefore, the answer for part A is:

`(x-(3)/(2))^2=(81)/(4)`

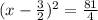
Part B.

Now, we need to solve the last result for x. Then, by applying square root to both sides, we have

`x-(3)/(2)=\pm\sqrt[]{(81)/(4)}`

which gives

`x-(3)/(2)=\pm(9)/(2)`

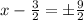
then, by adding 3/2 to both sides, we obtain

`x=(3)/(2)\pm(9)/(2)`

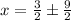
Then, we have 2 solutions,

`\begin{gathered} x=(3)/(2)+(9)/(2)=(12)/(2)=6 \\ \text{and} \\ x=(3)/(2)-(9)/(2)=(-6)/(2)=-3 \end{gathered}`

Therefore, the answer for part B is: -3, 6