Answer:
See graph below (graphs not numbered/lettered)
Step-by-step explanation:
Given:
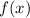
To find:
the graph of the function when it is -1/5f(x)
To determine the graph, we will pick points from the initial graph and apply the transformation -1/5f(x) to it
Using points: (-3, -5), (5, 3) and (-11, 3)
First, we apply a dilation with a scale factor of 1/5
We are multiplying the y coordinate by 1/5
(-3, -5) becomes (-3, -1)
(5, 3) becomes (5, 3/5)
(-11, 3) becomes (-11, 3/5)
Next, we will apply a reflection over the x axis. This will negate the y coordinate:
(-3, -1) becomes (-3, 1)
(5, 3/5) becomes (5, -3/5)
(-11, 3) becomes (-11, -3/5)
Plotting the points on the graph:
This graph in the option: