Question

A certain gas has a volume of 195 mL at 20°C and 1.00 atm. What is the its volume in mL at60°C and 600 mm Hg?

Answer 1

To solve this question we will assume that the moles of gas do not change and that the gas behaves like an ideal gas.

For an ideal gas we have that its behavior will be according to the following equation:

`PV=nRT`

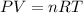
Where,

P is the pressure of the gas, in atm

V is the volume of the gas, in liters

n are the moles of the gas

T is the temperature of the gas, in Kelvin

R is a constant, 0.08206 atm.L/mol.K

We have for this gas two states, an initial state (1) and a final state (2), so for each state we can apply the ideal gas law, we will have:

Initial state:

`\begin{gathered} P_1V_1=nRT_1 \\ nR=(P_1V_1)/(T_1) \end{gathered}`

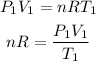
Final state:

`\begin{gathered} P_2V_2=nR_T_2 \\ nR=(P_2V_2)/(T_2) \end{gathered}`

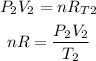
Since nR are constants, we can equate both equations:

`(P_1V_1)/(T_1)=(P_2V_2)/(T_2)`

For each state the conditions of pressure, volume and temperature will be:

P1=1.00atm

V1=195mL = 0.195L

T1=20°c = 293.15K

P2= 600mmHg=0.79atm

T2=60°C=333.15K

V2=Unknown

We clear V2 and replace the known data:

`V_2=(P_1V_1)/(T_1)*(T_2)/(P_2)`
`\begin{gathered} V_2=(1atm*0.195L)/(293.15K)*(333.15K)/(0.79atm) \\ V_2=(1*0.195*333.15)/(293.15*0.79)L \\ V_2=0.280L=280mL \end{gathered}`

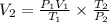
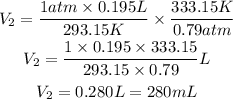
Answer: The final volume of the gas will be 280mL