Question

Find any domain restrictions on the given rational equation:

2/3x^2 + x/2 = x+5/x

Select all that apply.

A. x = -5
B. There are no restrictions on the domain.
C. X = 0
D. X = 2

Answer 1

It's a A. × = -5 and always there are no restrictions on the domain

Answer 2

The correct domain restriction is x=0, so option C is the correct answer.

To find domain restrictions on the given rational equation, we need to identify any values of x that would make the denominators equal to zero, as division by zero is undefined.

The given equation is:

`(2)/(3 x^2)+(x)/(2)=(x+5)/(x)`

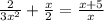
The denominators in the fractions are 3x², 2, and x. We need to check when each of these denominators would be equal to zero.

For 3x², the denominator will be zero when x=0 (because 0²=0).

For 2, the denominator is a constant and will never be zero.

For x, the denominator will be zero when x=0.

So, the values of x for which the denominators become zero are x=0.

Therefore, the correct domain restriction is: C. x=0