Question

Credit card A has an apr of 12.5% and an annual fee of $48 while credit card B has an apr of 15.49% and no annual fee. All else being equal which of these equations can be used to solve for the principal, p, the amount at which the cards offer the same deal over the course of a year? (Assume all interest is compounded monthly)

Answer 1

Step-by-step explanation: As we can see we are dealing with a monthly compound interest problem so we will need to use the following formula

Step 1: Once we have two different cards we will have two different equations following the formula above. Now let's identify the value of the variables for each card as follows.

- Card A: P = there is no information

r = 12.5%/100% = 0.125

t = 1 year

n = 12 monsths

- Card B: P = there is no information

r = 15.49%/100% = 0.1549

t = 1 year

n = 12 months

Step 2: There is still additional information we need to consider before building our expressions. Card A has an annual fee of $48 which means we need to add this value to our expression. On the other hand Card B has no annual fee which means our expression remains unchanged.

Step 3: Now we are able to substitute the values and build the expressions for Card A and B. Also, once we need to know "the amount at which the cards offer the same deal over the course of a year" we will make both equations equal as follows

`\begin{gathered} CardA=CardB \\ P(1+(0.125)/(12))^(12)+48=P(1+(0.154)/(12))^(12) \end{gathered}`

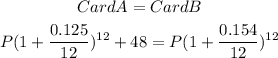
Final answer: As we can see above the final answer is the letter D.