Question

DeShawn has 120 feet of fencing for the pens. He likes his design because putting the two penstogether not only lets the pups safely play with each other, but also uses less fencing than if hemade both pens separate. Brilliant!

Answer 1

The optimal lenght of x and y are:

x = 30ft

y = 30ft

To solve this, we want to know the best lenght of x and y to get the maximum area.

Then, we know the perimeter of the pens: 120ft

The perimeter is the sum of all the sides:

`P=2x+2y`

Sinnce we have 2 sides of lenght x and 2 sides of length y

Also, we know how the area is calculated. Base times height:

`A=x\cdot y`

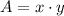
Now, we know the perimeter he has is 120ft. Then, we can replace P = 120ft and solve for y:

`\begin{gathered} P=120ft \\ 120ft=2x+2y \\ y=(120ft-2x)/(2) \\ y=60ft-x \end{gathered}`

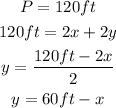
Now we can replace y in the area equation:

`\begin{gathered} \begin{cases}A=xy \\ y=60ft-x\end{cases} \\ A=x(60ft-x) \\ A=60ft\cdot x-x^2 \end{gathered}`

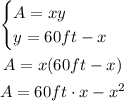
Here we have a function of the area respect x.

Then, we want to find the maximum area possible. We know that the zeroes of the derivative of a function is where we can find maximums and minimums.

Then let's derivate the function of the area:

`\begin{gathered} A(x)=60ft\cdot x-x^2 \\ A^(\prime)(x)=60ft-2x \end{gathered}`

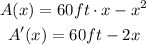
Now let's find the zero of the derivative:

`\begin{gathered} 0=60ft-2x \\ x=(-60ft)/(-2x) \\ x=30ft \end{gathered}`

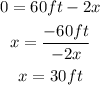
This is the value of x that maximizes the area with a perimeter of 120ft.

Now let's find the value of y:

`\begin{gathered} \begin{cases}x=30ft \\ y=60ft-x\end{cases} \\ y=60ft-30ft \\ y=30ft \end{gathered}`

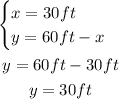
Then the maximum area is with a lenght of y = 30ft and x = 30ft.

This also tell us that the shape that maximizes the area of a 4 sided shape with a certain perimeter is a square.