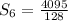Question

*I’m struggling with this calculus practice problem, I need it solved*

Answer 1

Given the series:

`40-10+2.5-0.625+...`

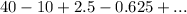
You need to remember that a Geometric Series has a Common Ratio, which is the factor between the terms.

In this case, the series is geometric, because every term is found by multiplying the previous one by:

`r=(r_2)/(r_1)=(-10)/(40)=-(1)/(4)`

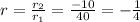
By definition, the sum of the first "n" terms of Geometric Series, can be calculated by using the following formula:

`S_n=(a_1(1-r^n))/(1-r)`

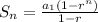
Where:

-The first term is:

`a_1`

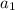
- The number of terms is "n".

- And "r" is the Common Ratio. This must be:

`r\\e1`

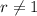
In this case, you can identify that:

`\begin{gathered} a_1=40 \\ \\ r=-(1)/(4) \\ \\ n=6 \end{gathered}`

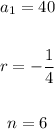
Therefore, substituting values into the formula and evaluating, you get:

`\begin{gathered} S_6=\frac{40_{}(1-(-(1)/(4))^6)}{1-(-(1)/(4))} \\ \\ S_6=\frac{40_{}(1-(-(1)/(4))^6)}{1+(1)/(4)} \end{gathered}`
`S_6=(4095)/(128)`

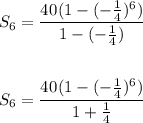
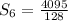
Hence, the answer is:

`S_6=(4095)/(128)`