Question

This table shows the relationship between the diameter, x, in inches, and the height, y, in feet, of trees in a national park. Diameter, x8.310.5111212.91416.317.317.918 Height, y70727575747877818081 What linear function best models the data in this table? Based on the model, what is the approximate height of a tree with a diameter of 22 inches? The data is best modeled by the function . Based on the linear model, the approximate height of a tree with a diameter of 22 inches is feet. The correlation coefficient for this model is 0.95, indicating that it a good model of the data.

Answer 1

From the table given in the question

We can compute the regression line equation as shown below

Using a graphing calculator, the equation is

`y_{}=1.04079x+61.9163`

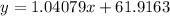
The data is modeled by

`y_{}=1.04079x+61.9163`

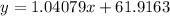
The approximate height of the tree whose diameter is 22 inches can be obtained by substituting x =22 into the equation.

We can also get the answer from the graph by also plotting x=22 on the same graph and then getting point of intersection.

From the graph, the height is 84.814 feet

Approximately, the height is about 85 feet.

Since the regression model is 0.95, this indicates that it is a positive and good model of the data.