Height:
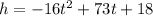
As we are asked the time when h = 91ft, then we have to set the equation to 91:

Now, we can solve for t by using the General Quadratic Equation:
![x_(1,2)=\frac{-b\pm\sqrt[]{b^2-4ac}}{2a}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/zndalnwykxb6ptn1p38z.png)
in which the variables represent the coefficients of a quadratic equation in the form:
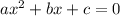
Therefore, we have to set our equation to 0:
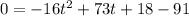
Simplifying:
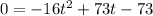
Thus, in our case:
• a = -16
,
• b = 73
,
• c = 73
Replacing these values in the formula:
![t_(1,2)=\frac{-73\pm\sqrt[]{73^2-4\cdot(-16)\cdot(-73)}}{2\cdot(-16)}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/ixk15jc69g3hh9j68l7l.png)
Simplifying:
![t_(1,2)=\frac{-73\pm\sqrt[]{657}}{-32}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/mdr0e3olfo1jj5djg2w1.png)
![t_1=\frac{-73+3\sqrt[]{73}}{-32}\approx3.08s](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/qtoowdzyvv5lj6shw8nd.png)
![t_2=\frac{-73-3\sqrt[]{73}}{-32}\approx1.48s](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/7br5vxk4grzesvi8nkoh.png)
As the object is thrown at 73ft/s, at 3 seconds it would be more or less three times higher than 73ft.
Answer: 1.48s