Question

Write a system of equations to describe the situation below, solve using any method, and fill in the blanks. The receivers for the Newton University football team are practicing running different routes on the field. They have to run a specific distance so that the quarterback knows exactly where to throw the ball. Wyatt ran 19 post routes and 21 slant routes, which meant he ran a total of 508 yards. Miguel ran 30 post routes and 20 slant routes, which equaled a total of 710 yards. How long is each route? A post route is yards long and a slant route is yards long.

Answer 1

Two players ran two different routes, player one ran 19 post routes and 21 slant routes and a total of 508 yards, similarly the player two ran 30 post routes and 20 slant routes and a total distance of 710 yards, following system of linear equations describe this situation:

`\begin{gathered} 19P+21S=508\rightarrow(1) \\ \\ 30P+20S=710\rightarrow(2) \end{gathered}`

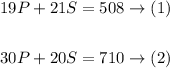
The solution to the (1) and (2) is as follows:

`\begin{gathered} S=x \\ P=y \\ \\ \therefore\rightarrow \\ 19y+21x=508 \\ 30y+20x=710 \end{gathered}`

The following plot shows the solution:

Therefore the answer is:

`\begin{gathered} S=x=7yd\rightarrow(I) \\ \\ P=y=19yd\rightarrow(II) \end{gathered}`

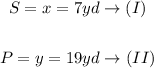
(I) is the length of the slant route and (II) is the length of the post route.