Question

a stone is projected at a cliff of height h with an initial speed of 42.0m/s directed at angle theta0=60.0 above the horizontal. The stone strikes at A, 5.50s after launching. Find (a) the height h of the cliff, (b) the speed of the stone just before impact at A, and (c) the maximum height H reached above the ground.

Answer 1

Height of the cliff: approximately
`51.7\; {\rm m}`.

Speed right before landing: approximately
`27.4\; {\rm m\cdot s^(-1)}`.

Maximum height reached: approximately
`67.4\; {\rm m}` above the ground (relative to the base of cliff.)

(Assumption: air resistance is negligible, and that
`g = 9.81\; {\rm m\cdot s^(-2)}`.)

Step-by-step explanation:

Let
`u` denote the velocity of the projectile at launch.

• Initial horizontal velocity:
  `u_(x) = u\, \cos(\theta) = 42.0\; {\rm m\cdot s^(-1)}\, \cos(60.0^(\circ)) = 21.0\; {\rm m\cdot s^(-1)}`.
• Initial vertical velocity:
  `u_(x) = u\, \sin(\theta) = (21.0\, √(3))\; {\rm m\cdot s^(-1)}`.

(a)

Under the assumptions, velocity in the vertical direction changes at a constant
`a_(y) = (-9.81)\; {\rm m\cdot s^(-2)}`. Velocity in the horizontal direction would be constant.

Apply the SUVAT equation
`x = (1/2)\, a\, t^(2) + u\, t` to find the vertical displacement after the
`t = 5.50\; {\rm s}` flight:

`\begin{aligned}x_(y) &= (1)/(2)\, a_(y)\, t^(2) + u_(y)\, t \\ &= (1)/(2)\, (-9.81)\, (5.50)^(2) + (21.0\, √(3))\, (5.50) \\ &\approx 51.7\; {\rm m}\end{aligned}`.

Thus, the cliff is approximately
`51.7\; {\rm m}` above the ground.

(b)

It is given that the flight took
`t = 5.50\; {\rm s}`. At a rate of
`a_(y) = (-9.81)\; {\rm m\cdot s^(-2)}`, vertical velocity would have changed by
`a_(y)\, t` during the flight. Hence, the vertical velocity right before landing would be:

`\begin{aligned}v_(y) &= u_(y) + a_(y)\, t \\ &= 21.0 √(3) + (-9.81)\, (5.50) \\ &\approx 17.582\; {\rm m\cdot s^(-1)} \end{aligned}`.

Under the assumptions, horizontal velocity would stay constant:
`v_(x) = u_(x) = 21.0\; {\rm m\cdot s^(-1)}`.

Apply the Pythagorean Theorem to find the overall velocity right before landing:

`\begin{aligned}v &= \sqrt{{v_(x)}^(2) + {v_(y)}^(2)} \\ &\approx \sqrt{21.0^(2) + (17.582)^(2)} \\ &\approx 27.4\; {\rm m\cdot s^(-1)}\end{aligned}`.

(c)

When height is maximized, vertical velocity would be
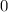`0`. Apply the SUVAT equation
`x = (v^(2) - u^(2)) / (2\, a)` to find the vertical displacement when vertical velocity is exactly
`0\; {\rm m\cdot s^(-1)}`:

`\begin{aligned}x_(y) &= \frac{{v_(y)}^(2) - {u_(y)}^(2)}{2\, a_(y)} \\ &= (0^(2) - (21.0 √(3))^(2))/(2\, (-9.81)) \\ &\approx 67.4\; {\rm m} \end{aligned}`.