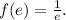Final Answer:
The function
 attains its absolute maximum value at ( x = e ), and the maximum value is
attains its absolute maximum value at ( x = e ), and the maximum value is
 .
.
Step-by-step explanation:
The critical points of the function
 occur where its derivative is zero or undefined. Taking the derivative using the quotient rule, we get:
occur where its derivative is zero or undefined. Taking the derivative using the quotient rule, we get:
![\[ f'(x) = (1 - ln(x))/(x^2) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/58b196biembl6p8hmw8li2ehiptuhh89bw.png)
Setting the numerator equal to zero gives
 and solving for ( x ) gives ( x = e ). Now, to determine whether this critical point is a maximum, minimum, or neither, we can use the second derivative test. The second derivative is:
and solving for ( x ) gives ( x = e ). Now, to determine whether this critical point is a maximum, minimum, or neither, we can use the second derivative test. The second derivative is:
![\[ f''(x) = (ln(x) - 3)/(x^3) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/nfqgyeiife6dl5avppxywa1gczbeig0bv7.png)
Substituting ( x = e ) into the second derivative, we find that
 . Since the second derivative is negative at ( x = e ), the function has a local maximum at this point.
. Since the second derivative is negative at ( x = e ), the function has a local maximum at this point.
To find the absolute maximum, we also need to consider the behavior of ( f(x) ) as ( x ) approaches zero and infinity. As ( x ) approaches zero, ( f(x) ) approaches negative infinity, and as \( x \) approaches infinity, ( f(x) ) approaches zero. Therefore, the absolute maximum value occurs at ( x = e ), and the maximum value is