Question

Question is attached. Show workings

Answer 1

First question(unnumbered):

`\mathrm{Choice \; (B) : \;\;(1)/(2)}`

Question 48

`\mathrm{Choice \;(C)\;\; (x, y) = \left(-1, (3)/(2)\right)}`

Question 49

`\mathrm{Choice \; (D)\;\;-1}`

Explanation:

The imaginary variable i is
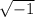`√(-1)` and
`i^2 = -1`

For the unnumbered first question we have

the equation

`(1)/(1-i)`

Multiply numerator and denominator by
`i + 1`

`\rightarrow (1\cdot \left(1+i\right))/(\left(1-i\right)\left(1+i\right))\\\\\\= (1 + i)/(1 - i^2) \;\;\;\;\;\;\;since \;(a +b)(a-b) = a^2 - b^2\\\\\textrm{Since $i^2 = -1$, the denominator becomes:}\\\\1-i^2 = 1 -(-1) = 2\\\\\\`

Therefore ,

`(1)/(1-i) = (1 + i)/(2)\\\\= (1)/(2) + (i)/(2)\\\\`

The real part is
`(1)/(2)` and the imaginary part is
`(i)/(2)`

Answer to first question is: )
`(1)/(2)`
-------------------------------------------------------------------------

#48

Solve
`(x, y)` for
`2y\:+\:xi\:=\:4+x-i` for
`$x,y\in\mathbb{R}$`

For the equation

`2y\:+\:xi\:=\:4+x-i`

Move the the x and i terms from the right to left side:

`2y + xi - x + i = 4\\\\2y - x +xi + i =4\\\\`

Since this works out to a real number, the imaginary part is 0

So

`xi + i = 0\\\\xi = -i\\\\x = (-i)/(i)\\\\x = -1`

and the real part

`2y - x = 4`

Substitute for x = -1 in the above to get

`2y -(-1) = 4\\\\2y + 1 = 4\\\\2y = 4-1\\\\2y = 3\\\\y = (3)/(2)\\\\`

`(x, y) = \left(-1, (3)/(2)\right)`
which is choice (C)

------------------------------------------------------------------------

#49

For
`$a,b\in\mathb{R}$`

`a+ib\:=\:\left(2-i\right)^2 \\\\`

Expand
`x(2-i)^2`

`= 2^2 - 2.2.i +i^2\\\\`

Since i² = -1 this works out to:

`4 - 4i -1`

`3 - 4i`

Therefore

`a + ib = 3 - 4i\\\\`

Equation real part on left to the real part on the right :

`a = 3`

Equation imaginary part on left to the imaginary part on the right :

`ib = 4i`

`b = -4`

`(a + b) = (3 +(-4))\\\\= 3 - 4 \\\\= -1\\\\`

This would be option (D)