Explanation:
firstly draw the triangle and label it. (you will find the picture down)
Now this remind us with the cosine rule. (you will find the picture down)
This rule says that the square of the given length of the side of a triangle is equal to the sum of the squares of the length of other sides minus twice their product and multiplied by the cosine of their included angle.
So using that rule we can say that:
 =
=
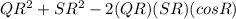
square root both sides, so you will have QS alone in one side.
QS =
![\sqrt{ [QR^(2) +SR^(2) - 2(QR)(SR)(cosR)]}](https://img.qammunity.org/2022/formulas/mathematics/middle-school/kxfkxmixkrkobfh5fcwnwoh4cz2hsdg7ck.png)
plug in the values we have and y=this will yield:
QS =
![\sqrt{ [11^(2) +16^(2) - 2(11)(16)(cos38)] }](https://img.qammunity.org/2022/formulas/mathematics/middle-school/3dxjax6bz2j1ne3f9vzucotldr0r6nq2x2.png) = 9.98099267 = 10
= 9.98099267 = 10