Question

If f(x) is a linear function, what is the value of n?

-4
-1
n
02
O
O 9
f(x)
-25
-10
20

Answer 1

Key Concepts

• Linear functions

Solving the Question

Determining the slope

In linear functions, there is a constant difference between the y-values on a table. This means that it increases or decreases by the same amount per every equal interval.

For instance: 2, 4, 6, 8 ⇒ Constant difference of 2 every 1 term

To find the constant difference for this set of values, determine the constant difference (y), and by what interval this difference occurs (x).

Looking at the first two rows of the table, we now know that for every increase in 3 in the x-values yields an increase of 15 in the y-values.

• difference between -4 and -1 = 3
• difference between -25 and -10 = 15

We can write it like a fraction, with difference in y on top and difference in x at the bottom:

`(15)/(3)` ⇒
`5`

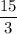Congratulations! We have now found the slope of the line.

Solving for n

Linear equations are typically organized in slope-intercept form:

`y=mx+b`

• m = slope
• b = y-intercept

Plug in our slope, and solve for the y-intercept by using one of the given points from the table:

`y=5x+b\\-10=5(-1)+b\\-10=-5+b\\b=-5`

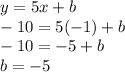
Plug this back into the equation:

`y=5x-5`

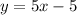
To find n, plug in 20 as y and solve:

`20=5n-5\\25=5n\\n=5`

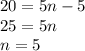
Answer

n = 5