Question

Complete the table for the arithmetic sequence.

Answer 1

Airthemetic Sequence : arithmetic sequence is a sequence of numbers such that the difference between the consecutive terms is constant.

It express as :

`a_n=a_1+(n-1)d`

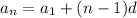
In the given question the 88 term is ( 25)

Substitute the value in the expression of n terms

`\begin{gathered} a_n=a_1+(n-1)d \\ \text{for : n =25, a}_n=88,a_1=(-8) \\ a_n=a_1+(n-1)d \\ 88=(-8)+(25-1)d \\ 88=-8+24d \\ 88+8\text{ =24d} \\ 24d=96 \\ d=(96)/(24) \\ d=4 \end{gathered}`

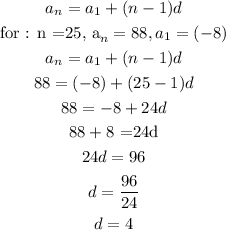
In the given Airthmetic sequence the constant difference, d = 4

Now for the position of term 8

`\begin{gathered} a_n=a_1+(n-1)d \\ \text{for a}_n=8,a_1=(-8),\text{ d =4} \\ 8=-8+(n-1)4 \\ 16=4(n-1) \\ 4=n-1 \\ n=5 \end{gathered}`

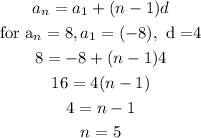
for n= 5 terms is 8

Now for the term of position 8:

`\begin{gathered} a_n=a_1+(n-1)d \\ \text{for n=8, a}_1=(-8),d=4 \\ a_n=-8+(8-1)4 \\ a_n=-8+7*4 \\ a_n=20 \end{gathered}`

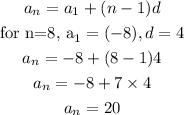
So, the term with position 8 is 20

Now for the position of term 36 :

`\begin{gathered} a_n=a_1+(n-1)d \\ \text{for :a}_n=36,a_1=(-8),\text{ d = 4} \\ 36=-8+(n-1)4 \\ 36+8=4(n-1) \\ 44=4(n-1) \\ n-1=(44)/(4) \\ n-1=11 \\ n=10 \end{gathered}`

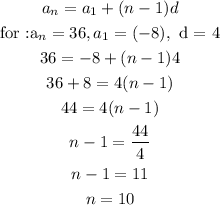
Thus, for n = 10, an = 36

Now, for the term of position 19

`\begin{gathered} a_n=a_1+(n-1)d \\ \text{for n=19, d=4, a}_1=(-8) \\ a_n=-8+(19-1)4 \\ a_n=-8+(18)4 \\ _{}a_n=-8+72 \\ a_n=64 \end{gathered}`

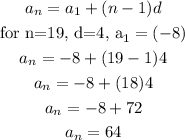
Thus at n = 19 the term i 64