Question

Determine whether the events described are overlapping or non-overlapping. Then find the either/or probability of the event.Randomly meeting a six -child family with either exactly one or exactly two girl children.

Answer 1

0.328175

Step-by-step explanation

The two events mentioned are:

• A: exactly 1 child is a girl

,

• B: exactly 2 children are girls

These events are not-overlapping because if exactly 1 of the 6 children is a girl, then there cannot be exactly two girls at the same time - and vice versa.

The probability that the family has exactly one girl or exactly two girls is,

`P(A\text{ }or\text{ }B)=P(A)+P(B)`

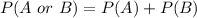
If there are 6 children in the family, the probability of randomly meeting a 6-child family with exactly 1 girl follows a binomial distribution, where the probability that a child is a girl is 0.5,

`P(A)=_6C_1\cdot0.5^1\cdot0.5^(6-1)=(6!)/(1!\cdot5!)\cdot0.5\cdot0.5^5=0.09375`

While the probability that the family has exactly 2 are girls is,

`P(B)=_6C_2\cdot0.5^2\cdot0.5^(6-2)=(6!)/(2!\cdot4!)\cdot0.5^2\cdot0.5^4=0.234375`

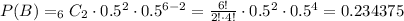
So, the probability that the family has exactly 1 girl or exactly 2 girls is,

`P(A\text{ }or\text{ }B)=0.09375+0.234375=0.328125`

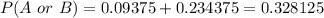
Hence, the probability of meeting a 6-child family with either exactly one or exactly two girls is 0.328175.