Recall that the sum of the interior angles of a convex polygon with n sides is:
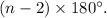
Therefore:
12) If the sum of the interior angles of a convex polygon is 3240 degrees, then we can set the following equation:

Dividing the above equation by 180 we get:

Adding 2 to the above equation we get:
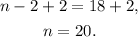
13) If the sum of the interior angles of a convex polygon is 6660 degrees, then we can set the following equation:
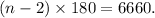
Dividing the above equation by 180 we get:

Adding 2 to the above equation we get:
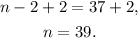
Answer:
12) 20.
13) 39.