Question

Write an equation of the perpendicular bisector of the segment with the endpoints G(3,7) and H(-1,-5).

Answer 1

`y=-(1)/(3)x+(4)/(3)`

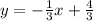
Step-by-step explanation

We want to find the equation of the perpendicular bisector of the segment with the endpoints G(3,7) and H(-1,-5).

Since the line is a bisector, it means that it passes through the midpoint of G and H.

Also, since it is perpendicular to the line with endpoints G and H, it means that the slope is the negative inverse of the slope of the line between the two points.

First, find the midpoint of the two points G and H:

`\begin{gathered} M=((x1+x2)/(2),(y1+y2)/(2)) \\ M=((3+(-1))/(2),(7+(-5))/(2)) \\ M=((2)/(2),(2)/(2)) \\ M=(1,1) \end{gathered}`

Next, find the slope of the line between points G and H:

`\begin{gathered} m=(y2-y1)/(x2-x1) \\ m=(-5-7)/(-1-3)=(-12)/(-4) \\ m=3 \end{gathered}`

Now, find the negative inverse:

`m_2=-(1)/(m_1)=-(1)/(3)`

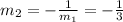
Find the equation of the line using the point-slope method:

`\begin{gathered} y-y1=m(x-x1) \\ y-1=-(1)/(3)(x-1) \\ y-1=-(1)/(3)x+(1)/(3) \\ y=-(1)/(3)x+(1)/(3)+1 \\ y=-(1)/(3)x+(4)/(3) \end{gathered}`

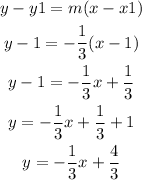
That is the equation of the line.