Question

A system of equations is shown below.

y = x^2 + 2x + 8
y = –4x
What is the smallest value of y in the solution set of the system?

Answer 1

y=8

Explanation:

Substitute y=-4x in the first equation to get
`-4x=x^2+2x+8.` This is equivalent to

`x^2+2x+8+4x=0\\x^2+6x+8=0\\(x+4)(x+2)=0\\x+4=0 \text{ OR } x+2=0\\x=-4 \text{ OR }x=-2`

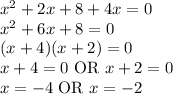
Therefore, the values of x in the system are x=-4 or x=-2.

Substitute x=-4 in y=-4x to get

`y=-4(-4)\\y=16.`

Substitute x=-2 in y=-4x to get

`y=-4(-2)\\y=8.`

Hence, the values of y in the system are y=16 or y=8. The smaller value is y=8.